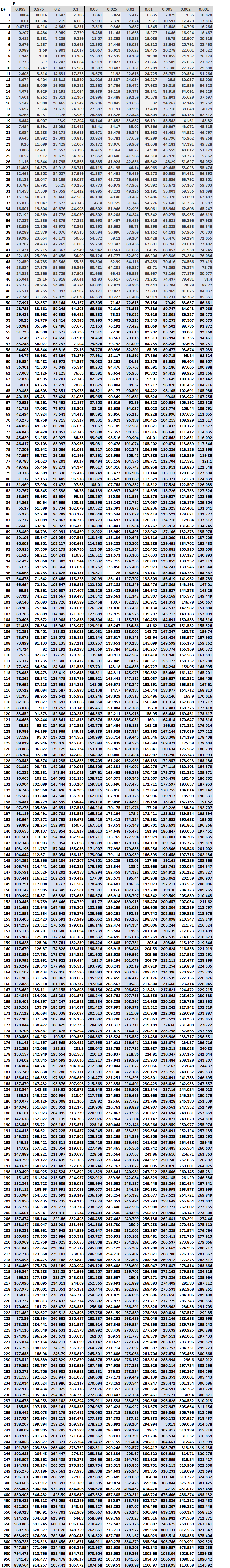
The Chi-square Distribution Table, also known as the Chi-square table, is a vital tool in statistical analysis for testing hypotheses about categorical data. Here’s an explanation of its purpose and usage:
What it shows:
- The Chi-square distribution table lists critical values for the Chi-square statistic (χ²), a statistical measure used to assess the discrepancy between observed and expected frequencies in categorical data.
- It features two main sections:
- One-tailed: Used for one-sided tests, where we are interested in the probability of a value falling in one tail of the distribution (e.g., higher than a certain value).
- Two-tailed: Used for two-sided tests, where we consider both tails of the distribution (e.g., significantly different from an expected value).
- Within each section, you’ll find:
- Degrees of freedom (df): Represents the number of independent categories in your data, affecting the shape of the Chi-square distribution.
- Significance level (α): Represents the probability of rejecting the null hypothesis (H0) when it’s actually true, typically 0.05 (5%) or 0.01 (1%).
- Critical values: These are specific thresholds for your calculated Chi-square statistic based on df and α.
How to use it:
-
Calculate your Chi-square statistic (χ²): This involves comparing observed and expected frequencies in your categorical data using a specific formula based on your chosen test (e.g., Chi-square test of independence, goodness-of-fit test).
-
Identify the appropriate section: One-tailed for one-sided tests, two-tailed for two-sided tests.
-
Locate the row with your degrees of freedom (df).
-
Find the column with your chosen significance level (α).
-
Compare your calculated Chi-square statistic to the critical value:
- Reject H0 if your Chi-square statistic is greater than or equal to the critical value. This indicates a statistically significant difference or association between the variables or a deviation from the expected distribution.
- Fail to reject H0 if your Chi-square statistic falls below the critical value. This suggests insufficient evidence for a significant difference or association.